Class 11 Physics Chapter Thermodynamics Notes

The branch of physics which deals with the study of transformation of heat energy into other forms of energy and vice-versa.

A thermodynamical system is said to be in thermal equilibrium when macroscopic variables (like pressure, volume, temperature, mass, composition etc) that characterise the system do not change with time.

Thermodynamical System

An assembly of an extremely large number of particles whose state can be expressed in terms of pressure, volume and temperature, is called thermodynamic system.

Thermodynamic system is classified into the following three systems

(i) Open System It exchange both energy and matter with surrounding.

(ii) Closed System It exchanges only energy (not matter) with surroundings.

(iii) Isolated System It exchanges neither energy nor matter with the surrounding.

A thermodynamic system is not always in equilibrium. For example, a gas allowed to expand freely against vacuum. Similary, a mixture of petrol vapour and air, when ignited by a spark is not an equilibrium state. Equilibrium is acquired eventually with time.

Thermodynamic Parameters or Coordinates or Variables

The state of thermodynamic system can be described by specifying pressure, volume, temperature, internal energy and number of moles, etc. These are called thermodynamic parameters or coordinates or variables.

Work done by a thermodynamic system is given by

W = p * ΔV

where p = pressure and ΔV = change in volume.

Work done by a thermodynamic system is equal to the area enclosed between the p-V curve and the volume axis

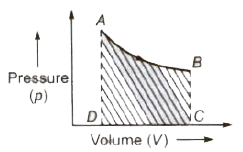
Work done in process A-B = area ABCDA

Work done by a thermodynamic system depends not only upon the initial and final states of the system but also depend upon the path followed in the process.

Work done by the Thermodynamic System is taken as

Positive → 4 as volume increases.

Negative → 4 as volume decreases.

Internal Energy (U)

The total energy possessed by any system due to molecular motion and molecular configuration, is called its internal energy.

Internal energy of a thermodynamic system depends on temperature. It is the characteristic property of the state of the system.

Zeroth Law of Thermodynamics

According to this law, two systems in thermal equilibrium with a third system separately are in thermal equilibrium with each other. Thus, if A and B are separately in equilibrium with C, that is if T_A = T_Cand T_B = T_C, then this implies that T_A= T_B i.e., the systems A and B are also in thermal equilibrium.

First Law of Thermodynamics

Heat given to a thermodynamic system (ΔQ) is partially utilized in doing work (ΔW) against the surrounding and the remaining part increases the internal energy (ΔU) of the system.

Therefore, ΔQ = ΔU + ΔW

First law of thermodynamics is a restatement of the principle conservation of energy.

In isothermal process, change in internal energy is zero (ΔU = 0).

Therefore, ΔQ = ΔW

In adiabatic process, no exchange of heat takes place, i.e., Δθ = O.

Therefore, ΔU = – ΔW

In adiabatic process, if gas expands, its internal energy and hence, temperature decreases and vice-versa.

In isochoric process, work done is zero, i.e., ΔW = 0, therefore

ΔQ = ΔU

Thermodynamic Processes

A thermodynamical process is said to take place when some changes’ occur in the state of a thermodynamic system i.e., the therrnodynamie parameters of the system change with time.

(i) Isothermal Process A process taking place in a thermodynamic system at constant temperature is called an isothermal process.

Isothermal processes are very slow processes.

These process follows Boyle’s law, according to which

pV = constant

From dU = nC_vdT as dT = 0 so dU = 0, i.e., internal energy is constant.

From first law of thermodynamic dQ = dW, i.e., heat given to the system is equal to the work done by system surroundings.

Work done W = 2.3026μRT l0g₁₀(V_f / V_i) = 2.3026μRT l0g₁₀(p_i / p_f)

where, μ = number of moles, R = ideal gas constant, T = absolute temperature and V_i V_f and P_i, P_f are initial volumes and pressures.

After differentiating P V = constant, we have

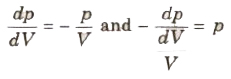
i.e., bulk modulus of gas in isothermal process, β = p.

P – V curve for this persons is a rectangular hyperbola

Examples

(a) Melting process is an isothermal change, because temperature of a substance remains constant during melting.

(b) Boiling process is also an isothermal operation.

(ii) Adiabatic Process A process taking place in a thermodynamic system for which there is no exchange of heat between the system and its surroundings.

Adiabatic processes are very fast processes.

These process follows Poisson’s law, according to which

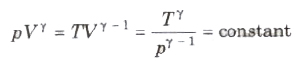
From dQ = nCdT, C_adi = 0 as dQ = 0, i.e., molar heat capacity for adiabatic process is zero.

From first law, dU = – dW, i.e., work done by the system is equal to decrease in internal energy. When a system expands adiabatically, work done is positive and hence internal energy decrease, i.e., the system cools down and vice-versa.

Work done in an adiabatic process is

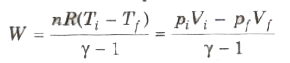
where T_i and T_f are initial and final temperatures. Examples

(a) Sudden compression or expansion of a gas in a container with perfectly non-conducting wall.

(b) Sudden bursting of the tube of a bicycle tyre.

(c) Propagation of sound waves in air and other gases.

(iii) Isobaric Process A process taking place in a thermodynamic system at constant pressure is called an isobaric process.

Molar heat capacity of the process is C_p and dQ = nC_pdT.

Internal energy dU = nC_v dT

From the first law of thermodynamics
dQ = dU + dW
dW = pdV = nRdT

Process equation is V / T = constant.

p- V curve is a straight line parallel to volume axis.

(iv) Isochoric Process A process taking place in a tlaermodynars system at constant volume is called an isochoric process.

dQ = nC_vdT, molar heat capacity for isochoric process is C_v.

Volume is constant, so dW = 0,

Process equation is p / T = constant

p- V curve is a straight line parallel to pressure axis.

(v) Cyclic Process When a thermodynamic system returns to . initial state after passing through several states, then it is called cyclic process.
Efficiency of the cycle is given by

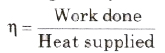
Work done by the cycle can be computed from area enclosed cycle on p- V curve.

Isothermal and Adiabatic Curves

The graph drawn between the pressure p and the volume V of a given mass of a gas for an isothermal process is called isothermal curve and for an adiabatic process it is called adiabatic curve .

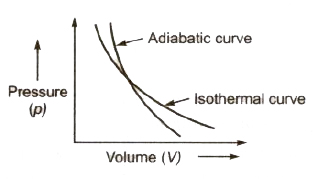
The slope of the adiabatic curve

= γ x the slope of the isothermal curve

Volume Elasticities of Gases

There are two types of volume elasticities of gases

(i) Isothermal modulus of elasticity E_S = p

(ii) Adiabatic modulus of elasticity E_T = γ p

Ratio between isothermal and adiabatic modulus

E_S / E_T = γ = C_p / C_V

where C_p and C_v are specific heats of gas at constant pressure and at constant volume.

For an isothermal process Δt = 0, therefore specific heat,

c = Δ θ / m Δt = &infi;

For an adiabatic process 119= 0, therefore specific heat,

c = 0 / m Δt = 0

Second Law of Thermodynamics

The second law of thermodynamics gives a fundamental limitation to the efficiency of a heat engine and the coefficient of performance of a refrigerator. It says that efficiency of a heat engine can never be unity (or 100%). This implies that heat released to the cold reservoir can never be made zero.

Kelvin’s Statement

It is impossible to obtain a continuous supply of work from a body by cooling it to a temperature below the coldest of its surroundings.

Clausius’ Statement

It is impossible to transfer heat from a lower temperature body to a higher temperature body without use of an extemal agency.

Planck’s Statement

It is impossible to construct a heat engine that will convert heat completely into work.

All these statements are equivalent as one can be obtained from the other.

Entropy

Entropy is a physical quantity that remains constant during a reversible adiabatic change.

Change in entropy is given by dS = δQ / T

Where, δQ = heat supplied to the system

and T = absolute temperature.

Entropy of a system never decreases, i.e., dS ≥ o.

Entropy of a system increases in an irreversible process

Heat Engine

A heat energy engine is a device which converts heat energy into mechanical energy.

A heat engine consists of three parts

(i) Source of heat at higher temperature

(ii) Working substance

(iii) Sink of heat at lower temperature

Thermal efficiency of a heat engine is given by

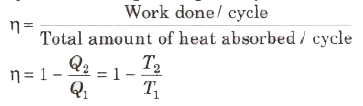
where Q₁ is heat absorbed from the source,

Q₂ is heat rejected to the sink and T₁ and T₂ are temperatures of source and sink.

Heat engine are of two types

(i) External Combustion Engine In this engine fuel is burnt a chamber outside the main body of the engine. e.g., steam engine. In practical life thermal efficiency of a steam engine varies from 12% to 16%.

(ii) Internal Combustion Engine In this engine. fuel is burnt inside the main body of the engine. e.g., petrol and diesel engine. In practical life thermal efficiency of a petrol engine is 26% and a diesel engine is 40%.

Carnot’s Cycle

Carnot devised an ideal cycle of operation for a heat engine, called Carnot’s cycle.

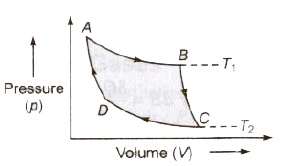
A Carnot’s cycle contains the following four processes

(i) Isothermal expansion (AB)

(ii) Adiabatic expansion (BO)

(iii) Isothermal compression (CD)

(iv) Adiabatic compression (DA)

The net work done per cycle by the engine is numerically equal to the area of the loop representing the Carnot’s cycle .

After doing the calculations for different processes we can show that

[Efficiency of Carnot engine is maximum (not 1000/0) for given temperatures T₁ and T₂. But still Carnot engine is not a practical
engine because many ideal situations have been assumed while designing this engine which can practically not be obtained.]

Refrigerator or Heat Pump

A refrigerator or heat pump is a device used for cooling things. It absorb heat from sink at lower temperature and reject a larger amount of heat to source at higher temperature.

Coefficient of performance of refrigerator is given by

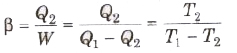
where Q₂ is heat absorbed from the sink, Q₁ is heat rejected to source and T₁ and T₂ are temperatures of source and sink.

Relation between efficiency (η) and coefficient of performance (β)